Main Staff		Prof. Jun OTA
Sub Staff		Prof. Tamio ARAI
Students		Ryusuke CHIBA，　Takayuki HARA
Contents		In this study, we present a method of an integrated design for automated guided vehicle (AGV) systems. Recently, a material handling system using AGVs (Automated Guided Vehicles) have been used in manufacturing factories. AGVs are programmable material handling equipment. These vehicles circulate on a guide-paths network and transport materials among storages and machines in the factories (see Fig.1). Keywords: AGV, Integrated Design, Transporter Routing, Flow Path Network
Abstract of Projects		Generally, the design problem for AGV systems divides into two sub-problems; a flow path design problem and a transporter routing design problem The flow path design problem is the issue how to put guides on the floor of factory. The transporter routing design problem is the issue how to operate AGVs. In this study, we solve these two problems. It is better for the workers that there are not many aisles of AGVs at these AGV systems, because AGVs and workers work together in the factory. 　It is important that these two problems influence each other. Therefore, they should not be dealt independently. For the high perform AGV systems, integrated design process of three design problems is needed. In this study, we propose a method of an integrated design for AGV systems. 　For this purpose, we propose the method that takes three steps. 1) We describe the transporter routing as parameters and design them by Genetic Algorithm. 2) We describe the flow path as connections between cells and design them by Genetic Algorithm. 3) The 2 Genetic Algorithm are cooperated by co-evolution and we provide the integrated design method. 　In 1), the parameters are their quality and quantity of the information when we plan actions of AGVs. The information is the perception of other AGVs. In 2), the environment are divided cells and genes in GA are the connections between them. In 3), a partner strategy of co-evolution should be solved. We solve them using "best vs. all". This strategy is that the partner in evolution process is most valuable individual. 　With our method, we obtain the set of transporter routing and flow path by simulation. Fig.2 shows the result in 4 AGVs, 3 storages (blue and vertical lines) and 2 machines (red and blue and horizontal lines). Then we set the frequency of tasks to be lower, and construct the AGV system. The result is shown in Fig.3. The flow path is simple and shortage compared with Fig.2. AGV Systems adapted to conditions can be constructed. 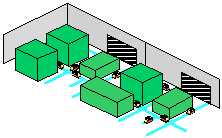Fig.1 AGV manufacturing syste 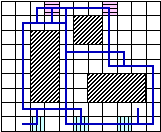　　　　 Fig.2 Flow path network in 7 steps　　　Fig. 3 Flow path network in 12 steps	Movie
			Movie Transport Simulation (Animation GIF)
			-
			-
			-
			※Each file size is less 1[Mbyte]．
			Go to PDF

Back to Research Page of Ota Lab.

Back to Top Page of Ota Lab.